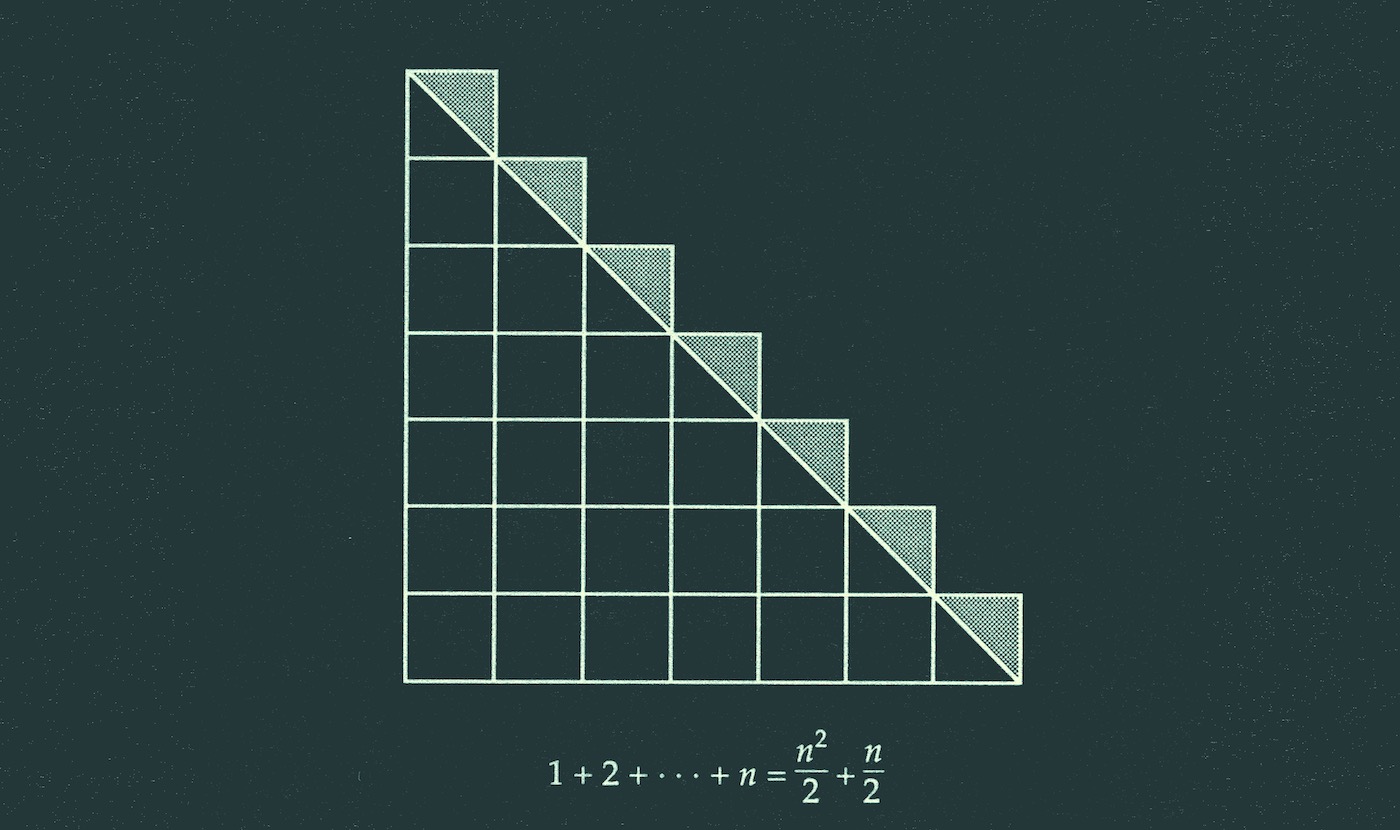
SAV du DL n°2 de Mathématiques
\(\newcommand{\eps}{\varepsilon}
\newcommand{\llbracket}{[\![}
\newcommand{\rrbracket}{]\!]}
\newcommand{\D}{\mathrm{D}}
\newcommand{\C}{\mathbb{C}}
\newcommand{\Q}{\mathbb{Q}}
\newcommand{\R}{\mathbb{R}}
\newcommand{\U}{\mathbb{U}}
\newcommand{\K}{\mathbb{K}}
\newcommand{\N}{\mathbb{N}}
\newcommand{\M}{\mathrm{M}}
\newcommand{\DL}{\mathrm{DL}}
\newcommand{\rg}{\mathrm{rg}\,}
\newcommand{\id}{\mathrm{id}}
\newcommand{\GL}{\mathrm{GL}}
\newcommand{\card}{\mathrm{Card}\,}
\newcommand{\Det}{\mathrm{Det}}
\newcommand{\union}{\cup}
\renewcommand{\Im}{\mathrm{Im}\,}
\renewcommand{\Re}{\mathrm{Re}\,}
\newcommand{\Ker}{\mathrm{Ker}\,}
\newcommand{\vect}{\mathrm{vect}}
\newcommand{\inter}{\cap}
\newcommand{\ch}{\mathrm{ch}\,}
\newcommand{\sh}{\mathrm{sh}\,}
\renewcommand{\th}{\mathrm{th}\,}
\newcommand{\argch}{\mathrm{argch}\,}
\newcommand{\argsh}{\mathrm{argsh}\,}
\newcommand{\argth}{\mathrm{argth}\,}
\newcommand{\Z}{\mathbb{Z}}
\newcommand{\mfrac}[2]{\genfrac{}{}{0pt}{}{#1}{#2}}
\newcommand{\cotan}{\mathrm{cotan}\,}
\newcommand{\tr}{\mathrm{Tr}\,}
\)
Le DL n°02 porte sur les calculs de sommes, les raisonnements par récurrence !
Comments (1)
Laisser un commentaire
Vous devez vous connecter pour publier un commentaire.

Le SAV du DL2 est ouvert …